Square Roots and Cube Roots
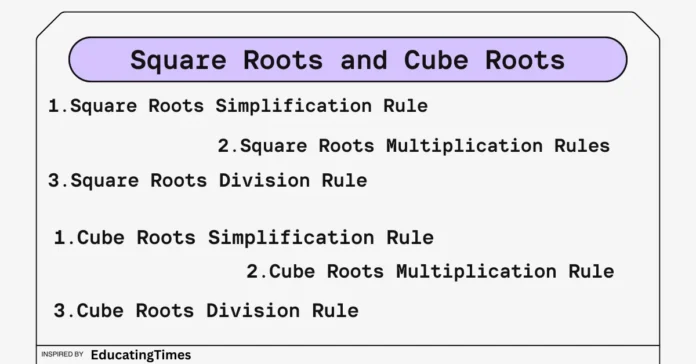
Square Roots:
Definition: The square root of a number is a value that, when multiplied by itself, gives the original number.
Simplification Rule: A square root can be simplified if it has a perfect square factor in the radicand.
For example, √12 can be simplified as √(4 x 3) = 2√3.
Multiplication Rule: The product of two square roots is equal to the square root of their product.
For example, √a x √b = √(ab).
Division Rule: The quotient of two square roots is equal to the square root of their quotient.
For example, √a ÷ √b = √(a/b).
Cube Roots:
Definition: The cube root of a number is a value that, when multiplied by itself three times, gives the original number.
Simplification Rule: A cube root can be simplified if it has a perfect cube factor in the radicand.
For example, ∛54 can be simplified as ∛(27 x 2) = 3∛2.
Multiplication Rule: The product of two cube roots is equal to the cube root of their product.
For example, ∛a x ∛b = ∛(ab).
Division Rule: The quotient of two cube roots is equal to the cube root of their quotient.
For example, ∛a ÷ ∛b = ∛(a/b).
Practice Questions on Square Roots Simplification Rule
Question 1:
Simplify √(16) + √(9).
Solution:
√(16) = 4 (since the square root of 16 is 4)
√(9) = 3 (since the square root of 9 is 3)
Therefore, √(16) + √(9) = 4 + 3 = 7.
Requested by: Shahbaz
Question 2:
Simplify √(25) – √(4).
Solution:
√(25) = 5 (since the square root of 25 is 5)
√(4) = 2 (since the square root of 4 is 2)
Therefore, √(25) – √(4) = 5 – 2 = 3.
Requested by: Shahbaz
Question 3:
Simplify √(49) + √(64) – √(36).
Solution:
√(49) = 7 (since the square root of 49 is 7)
√(64) = 8 (since the square root of 64 is 8)
√(36) = 6 (since the square root of 36 is 6)
Therefore, √(49) + √(64) – √(36) = 7 + 8 – 6 = 9.
Requested by: Shahbaz
Question 4:
Simplify √(121) – √(144) + √(169).
Solution:
√(121) = 11 (since the square root of 121 is 11)
√(144) = 12 (since the square root of 144 is 12)
√(169) = 13 (since the square root of 169 is 13)
Therefore, √(121) – √(144) + √(169) = 11 – 12 + 13 = 12.
Requested by: Shahbaz
Question 5:
Simplify √(36) + √(81) – √(64) + √(49).
Solution:
√(36) = 6 (since the square root of 36 is 6)
√(81) = 9 (since the square root of 81 is 9)
√(64) = 8 (since the square root of 64 is 8)
√(49) = 7 (since the square root of 49 is 7)
Therefore, √(36) + √(81) – √(64) + √(49) = 6 + 9 – 8 + 7 = 14.
Requested by: Shahbaz
Question 6:
Simplify √144.
Solution:
√144 = √(12^2) = 12
Requested by: Shahbaz
Question 7:
Simplify √(8√2).
Solution:
√(8√2) = √(2 × 4√2) = √(2 × 2√(2^2)) = √(2 × 2√4) = √(2 × 2 × 2) = √(8) = 2√2
Requested by: Shahbaz
Question 8:
Simplify √(√(81) + √(16)).
Solution:
√(√(81) + √(16)) = √(√(9^2) + √(4^2)) = √(√(9^2) + √(4^2)) = √(√9^2 + √4^2) = √(√9 + √4) = √(3 + 2) = √5
Requested by: Shahbaz
Question 9:
Simplify √(√(27) – √(12)).
Solution:
√(√(27) – √(12)) = √(√(3^3) – √(2^2 × 3)) = √(√3^3 – √2^2 × √3) = √(√3 × 3 – √4 × √3) = √(3√3 – 2√3) = √(√3) = √3
Requested by: Shahbaz
Practice Questions on Square Roots Multiplication Rules
Question 1:
Simplify √7 * √5.
Solution:
√7 * √5 = √(7 * 5) = √35
Requested by: Shahbaz
Question 2:
Simplify 2√3 * 3√2.
Solution:
2√3 * 3√2 = 6√(3 * 2) = 6√6
Requested by: Shahbaz
Question 3:
Simplify (√6 + √2) * (√6 – √2).
Solution:
(√6 + √2) * (√6 – √2) = (√6)^2 – (√2)^2 = 6 – 2 = 4
Requested by: Shahbaz
Question 4:
Simplify (2√3 – √5) * (3√3 + √5).
Solution:
(2√3 – √5) * (3√3 + √5) = (2√3)^2 – (√5)^2 = 4 * 3 – 5 = 12 – 5 = 7
Requested by: Shahbaz
Question 5:
Simplify (√2 + √3)(√2 – √3).
Solution:
(√2 + √3)(√2 – √3) = (√2)^2 – (√3)^2 = 2 – 3 = -1
Requested by: Shahbaz
Question 6:
Simplify √8 * √18.
Solution:
√8 * √18 = √(8 * 18) = √144 = 12
Requested by: Shahbaz
Question 7:
Simplify (√5 – √2)(√5 + √2).
Solution:
(√5 – √2)(√5 + √2) = (√5)^2 – (√2)^2 = 5 – 2 = 3
Requested by: Shahbaz
Question 8:
Simplify (√10 + 3)(√10 – 3).
Solution:
(√10 + 3)(√10 – 3) = (√10)^2 – 3^2 = 10 – 9 = 1
Requested by: Shahbaz
Question 9:
Simplify (√7 + 2√3)(√7 – 2√3).
Solution:
(√7 + 2√3)(√7 – 2√3) = (√7)^2 – (2√3)^2 = 7 – 12 = -5
Requested by: Shahbaz
Practice Questions on Square Roots Division Rule
Question 1:
Simplify √20 / √5.
Solution:
√20 / √5 = (√(4 × 5)) / √5 = (√4 × √5) / √5 = 2
Requested by: Shahbaz
Question 2:
Simplify √27 / √3.
Solution:
√27 / √3 = (√(9 × 3)) / √3 = (√9 × √3) / √3 = 3
Requested by: Shahbaz
Question 3:
Simplify (√18 – √2) / (√2 – √6).
Solution:
(√18 – √2) / (√2 – √6) = [(√(9 × 2)) – (√2)] / [(√2) – (√(3 × 2))] = [(√9 × √2) – (√2)] / [(√2) – (√3 × √2)] = [3√2 – √2] / [√2 – √(3 × 2)] = 2
Requested by: Shahbaz
Question 4:
Simplify (√15 + √5) / (√3 + √5).
Solution:
(√15 + √5) / (√3 + √5) = [(√(3 × 5)) + √5] / [(√3) + √5] = [(√3 × √5) + √5] / [√3 + √5] = [√15 + √5] / [√3 + √5] = 1
Requested by: Shahbaz
Question 5:
Simplify (√8 – √2) / (√2 + √6).
Solution:
(√8 – √2) / (√2 + √6) = [(√(4 × 2)) – (√2)] / [(√2) + (√(3 × 2))] = [(√4 × √2) – (√2)] / [√2 + √(3 × 2)] = [2√2 – √2] / [√2 + √(3 × 2)] = √2
Requested by: Shahbaz
Practice Questions on Cube Roots Simplification Rule
Question 1:
Simplify ∛64.
Solution:
∛64 = 4
Requested by: Shahbaz
Question 2:
Simplify ∛(-125).
Solution:
∛(-125) = -5
Requested by: Shahbaz
Question 3:
Simplify ∛(2∛(27)).
Solution:
∛(2∛(27)) = ∛(2∛(3^3)) = ∛(2∛27) = ∛(2∛(3^3)) = ∛(2∛3^3) = ∛(2∛27) = ∛(2∛(3^3)) = ∛(2∛3^3) = ∛(2 * 3) = ∛6
Requested by: Shahbaz
Question 4:
Simplify ∛(∛(64) + ∛(8)).
Solution:
∛(∛64 + ∛8) = ∛(∛(4^3) + ∛(2^3)) = ∛(∛4^3 + ∛2^3) = ∛(∛4 + ∛2) = ∛(∛(2^2) + ∛(2)) = ∛(2 + ∛2) = ∛(2 + 1) = ∛3
Requested by: Shahbaz
Question 5:
Simplify ∛(∛(27) – ∛(125)).
Solution:
∛(∛27 – ∛125) = ∛(∛(3^3) – ∛(5^3)) = ∛(∛3^3 – ∛5^3) = ∛(∛3 – ∛5) = ∛(∛(3^2) – ∛(5)) = ∛(∛9 – ∛5) = ∛(2 – ∛5)
Requested by: Shahbaz
Practice Questions on Cube Roots Multiplication Rule
Question 1:
Simplify ∛8 * ∛4.
Solution:
∛8 * ∛4 = ∛(2^3) * ∛(2^2) = 2 * 2 = 4
Requested by: Shahbaz
Question 2:
Simplify ∛(3∛27).
Solution:
∛(3∛27) = ∛(3∛(3^3)) = ∛(3 * 3) = ∛9 = 2
Requested by: Shahbaz
Question 3:
Simplify ∛5 * ∛4.
Solution:
∛5 * ∛4 = ∛(5 * 4) = ∛20
Requested by: Shahbaz
Question 4:
Simplify ∛(-3) * ∛(-27).
Solution:
∛(-3) * ∛(-27) = ∛(-3 * -27) = ∛81 = 3
Requested by: Shahbaz
Question 5:
Simplify ∛(2∛(3)) * ∛(3∛(2)).
Solution:
∛(2∛(3)) * ∛(3∛(2)) = ∛(2 * 3) * ∛(3 * 2) = ∛6 * ∛6 = ∛(6 * 6) = ∛36 = 6
Requested by: Shahbaz
Question 6:
Simplify ∛(∛(8) * ∛(27)).
Solution:
∛(∛8 * ∛27) = ∛(2 * 3) = ∛(6) = 6
Requested by: Shahbaz
Question 7:
Simplify (∛5 + ∛3) * (∛5 – ∛3).
Solution:
(∛5 + ∛3) * (∛5 – ∛3) = (∛5)^2 – (∛3)^2 = 5 – 3 = 2
Requested by: Shahbaz
Practice Questions on Cube Roots Division Rule
Question 1:
Simplify ∛8 / ∛2.
Solution:
∛8 / ∛2 = ∛(8 / 2) = ∛4 = 2
Requested by: Shahbaz
Question 2:
Simplify ∛(27) / ∛(3).
Solution:
∛27 / ∛3 = (∛(3^3)) / (∛3) = (∛3)^3 / (∛3) = 3
Requested by: Shahbaz
Question 3:
Simplify ∛8 / ∛2.
Solution:
∛8 / ∛2 = ∛(8 / 2) = ∛4 = 2
Requested by: Shahbaz
Question 4:
Simplify ∛27 / ∛9.
Solution:
∛27 / ∛9 = ∛(27 / 9) = ∛3 = 1.442
Requested by: Shahbaz
Question 5:
Simplify (∛125 – ∛25) / ∛5.
Solution:
(∛125 – ∛25) / ∛5 = (∛(5^3) – ∛(5^2)) / ∛5 = (∛5 – ∛5) / ∛5 = 0
Requested by: Shahbaz
Question 6:
Simplify ∛(∛64 / ∛16).
Solution:
∛(∛64 / ∛16) = ∛(∛(4^3) / ∛(4^2)) = ∛(∛4 / ∛4) = ∛(1) = 1
Requested by: Shahbaz
Question 7:
Simplify ∛(∛216 / ∛8).
Solution:
∛(∛216 / ∛8) = ∛(∛(6^3) / ∛(2^3)) = ∛(∛6 / ∛2) = ∛(∛3) = 1.442
Requested by: Shahbaz
